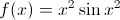Call Now to Set Up Tutoring:

(888) 888-0446

AP Calculus AB : An intuitive understanding of continuity

Study concepts, example questions & explanations for AP Calculus AB

Create An Account Create Tests & Flashcards

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 :Continunity As A Property Of Functions

Is the following piecewise function continuous for all? If not, state where it is discontinuous.

$f(x) = \left{\begin{Bmatrix} x^2+3 & 3 < x \\ 5x-3 & 3 \leq x < 5 \\ 11(x-3) &x \geq 5 \\ \end{matrix}\right.}$

Possible Answers:

No. The function is not continuous at x = 5 .

Yes. The function is continuous at all.

No. The function is not continuous at both x = 3 and x = 5 .

No. There are sharp turns at x = 3 and x = 5 .

No. The function is not continuous at x = 3 .

Correct answer:

Yes. The function is continuous at all.

Explanation:

To check if the piecewise function is continuous, all we need to do is check that the values at 3 and 5 line up.

At x =3 , this means checking that x^2 + 3 and 5x -3 have the same value. More formally, we are checking to see that $f(3) = \lim_{x\rightarrow3^-} f(x)$ , as to be continuous at a point, a function's left and right limits must both equal the function value at that point.

Plugging 3 into both, we see that both of them are 12 at x =3 . Thus, they meet up smoothly.

Next, for x = 5 , we have 5x -3 and 11(x-3) . Plugging in 5, we get 22 for both equations.

As all three equations are polynomials, we know they will be continuous everywhere else, and they meet up smoothly at the piecewise bounds, thus ensuring that the function is continuous everywhere.

Note, therearesharp turns at x = 3 and x = 5 , but this only means the function isn't differentiable at these points -- we're only concerned with continuity, which is if the equations meet up. Thus, the function is continuous.

Report an Error

Example Question #1 :An Intuitive Understanding Of Continuity

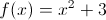
$f(x)={x^{2}+3}$ when x<3 and

$f(x)={7x-3}$ when $x\geq 3$

At x=6 the funciton described above is:

Possible Answers:

neither differentiable or continuous

differentiable but not continuous

both continuous and diffentiable

continuous but not differentiable

undefined

Correct answer:

both continuous and diffentiable

Explanation:

The answer is both.

If graphed the student will see that the two graphs are continuous at x=6 . There is no gap in the graph or no uneven transitions. If the graph is continuous then it is differentiable so it must be both.

Report an Error

Example Question #61 :Derivative At A Point

Which of the following functions contains a removeable discontinuity?

Possible Answers:

$f(x)=\frac{x+1}{1+x^{2}}$

$f(x)=1-(\ln x)^{2}$

$f(x)=\frac{1+x^{3}}{1+x}$

$f(x)=\frac{x}{1-x^{2}}$

$f(x)=x^{2}\sin x^{2}$

Correct answer:

$f(x)=\frac{1+x^{3}}{1+x}$

Explanation:

A removeable discontinuity occurs whenever there is a hole in a graph that could be fixed (or "removed") by filling in a single point. Put another way, if there is a removeable discontinuity at x=a , then the limit asapproachesexists, but the value of f(a) does not.

For example, the function $f(x)=\frac{1+x^3}{1+x}$ contains a removeable discontinuity at x=-1 . Notice that we could simplify f(x) as follows:

$f(x)=\frac{1+x^3}{1+x}=\frac{(1+x)(x^2-x+1)}{1+x}=x^{2}-x+1$ , where $x\neq -1$ .

Thus, we could say that $\lim_{x\rightarrow -1}\frac{1+x^3}{1+x}=\lim_{x\rightarrow -1}x^2-x+1=(-1)^2-(-1)+1=3$ .

As we can see, the limit of f(x) exists at x=-1 , even though f(-1) is undefined.

What this means is that f(x) will look just like the parabola with the equation $x^{2}-x+1$ EXCEPT when x=-1 , where there will be a hole in the graph. However, if we were to just define f(-1)=3 , then we could essentially "remove" this discontinuity. Therefore, we can say that there is a removeable discontinuty at x=-1 .

The functions

$f(x)=\frac{x}{1-x^{2}}$ , and

$f(x)=1-(\ln x)^{2}$

have discontinuities, but these discontinuities occur as vertical asymptotes, not holes, and thus are not considered removeable.

The functions

$f(x)=x^{2}\sin x^{2}$ and $f(x)=\frac{x+1}{1+x^{2}}$ are continuous over all the real values of; they have no discontinuities of any kind.

The answer is

$f(x)=\frac{1+x^{3}}{1+x}$ .

Report an Error

View AP Calculus AB Tutors

Steven
Certified Tutor

University of Louisville, Bachelor of Science, Mechanical Engineering. University of Phoenix-Louisville Campus, Master of Eng...

View AP Calculus AB Tutors

Samuel
Certified Tutor

Ohio State University-Main Campus, Bachelor of Science, Computer Science.

View AP Calculus AB Tutors

Pankaj
Certified Tutor

University of Delhi, Electrical Engineer, Electrical Engineering. Columbia University in the City of New York, Master of Arts...

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

GRE Tutors in Houston,English Tutors in Miami,考试辅导in Chicago,Chemistry Tutors in Miami,GRE Tutors in Chicago,MCAT Tutors in Philadelphia,Algebra Tutors in Houston,Physics Tutors in Philadelphia,English Tutors in Boston,Chemistry Tutors in San Francisco-Bay Area

Popular Courses & Classes

ISEE Courses & Classes in San Diego,GMAT Courses & Classes in Chicago,坐在课程s & Classes in Philadelphia,GMAT Courses & Classes in Houston,GRE Courses & Classes in Miami,GRE Courses & Classes in Atlanta,ACT Courses & Classes in Philadelphia,坐在课程s & Classes in Denver,Spanish Courses & Classes in Dallas Fort Worth,LSAT Courses & Classes in Philadelphia

Popular Test Prep

SSAT Test Prep in San Francisco-Bay Area,SAT Test Prep in Atlanta,SSAT Test Prep in Chicago,MCAT Test Prep in Chicago,GMAT Test Prep in Philadelphia,LSAT Test Prep in Phoenix,GRE Test Prep in Denver,GMAT Test Prep in New York City,SAT Test Prep in San Diego,GMAT Test Prep in Washington DC

DMCA Complaint

如果你认为可用的内容the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

*Full legal name

Company name

*Phone number

*Email address

Address

*Address1

Address2

*City

*State

*Zipcode

*Country

Complaint Details

*URL of copyrighted work (where your original material is located)

*Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

*Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Calculus AB : An intuitive understanding of continuity

Study concepts, example questions & explanations for AP Calculus AB

All AP Calculus AB Resources

Example Questions

Example Question #1 :Continunity As A Property Of Functions

Example Question #1 :An Intuitive Understanding Of Continuity

Example Question #61 :Derivative At A Point

All AP Calculus AB Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Find the Best Tutors

Email address:
Your name:

Email address:
Your name:
Feedback: